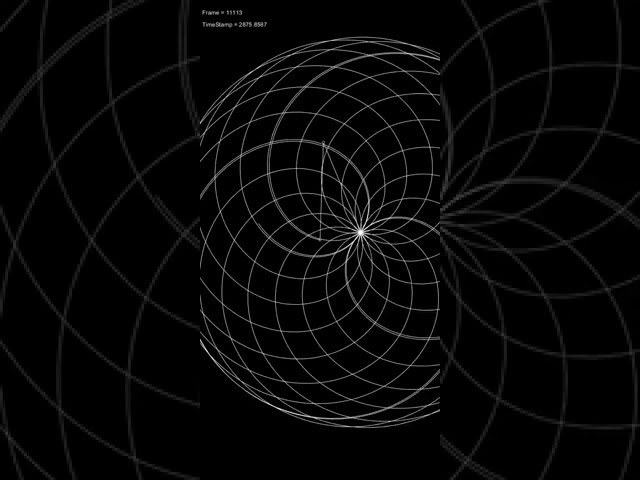
When they say the number π, it’s a dumb number:
BlueJakk
A few days ago it was pi day. The mysterious dumb number that can be approximated by dividing the circumference of any circle by its diameter: 3.14
For this reason, the fourteenth day of the third month of the year is Pi Day
I wrote the following text about the above video five months ago, but I didn’t publish it:
The above video has been reposted many times on the internet in the last 48 hours: youtube
A dumb number is a number that cannot be written as a ratio of two integers. For example, radical 2: wikipedia
That is, there are no two integers that if we divide them together, we can find the number. No matter how much they read and calculate it, they still cannot reach the bottom.
It seems that by 2020, they could calculate it with an accuracy of up to 12.8 trillion decimal digits, and with the advancement of computers in 2021, they could calculate it with an accuracy of up to 62.8 trillion decimal digits:
The least accurate calculation for pi is to consider it equal to 3.
After that, the most basic calculations are the following fractions that I wrote up to 11 decimal places:
22 / 7 = 3.14285714286
333 / 106 = 3.14150943396
355 / 113 = 3.14159292035
52163 / 16604 = 3.14159238738
103993 / 33102 = 3.14159265301
245850922 / 78256779 = 3.14159265359
In the above video, the equation of the graph to be drawn is written below it.
What does this show?
Every time the drawing point passes by the starting point with a difference, it shows the accuracy of one of the above calculations. Every time it passes by the starting point, the distance decreases, but it never reaches it because you can never accurately calculate a dumb number.
It seems that the second 0:11 of the above video, the distance between the drawing point and the starting point corresponds to the time when we consider pi as an integer of 3.
The 0:48 second of the video that zooms in and shows it passing by with a small distance is related to the precision of the fraction 7/22. It seems that the time 01:46 of the video is related to the accuracy of the fraction 333/106, and the time 02:14 of the video shows the accuracy of calculating the fraction 355/113, which is more accurate and passes the starting point with a shorter distance and continues like this.